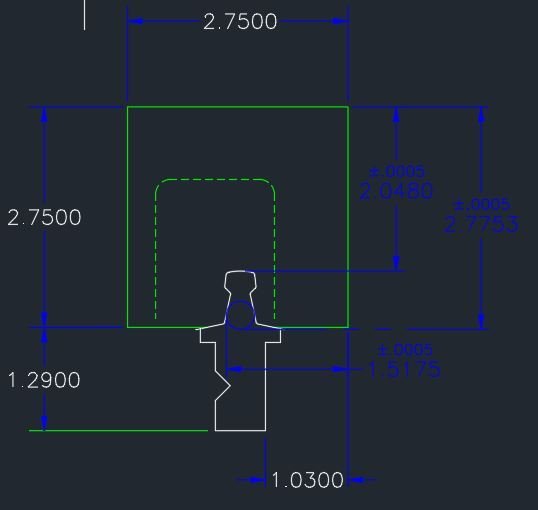
Attached is a print snippet. How can I evaluate the 2.7753 and 1.5175 dimensions?
Your Products have been synced, click here to refresh

Hello
Evaluate the distance from the upper planen to centerpoint and add half the center circle diameter.
Evaluate the distance from the rigt plane to P2.
Hope this helps.
I was thinking there might be a command for this such as 3McdCirCir2, except one element would be an axis. There is a command MCDCIAX. but this is to the center of the circle.
Hello.
What i can see on the drawing the 1.5175 distance is to the tangency point.
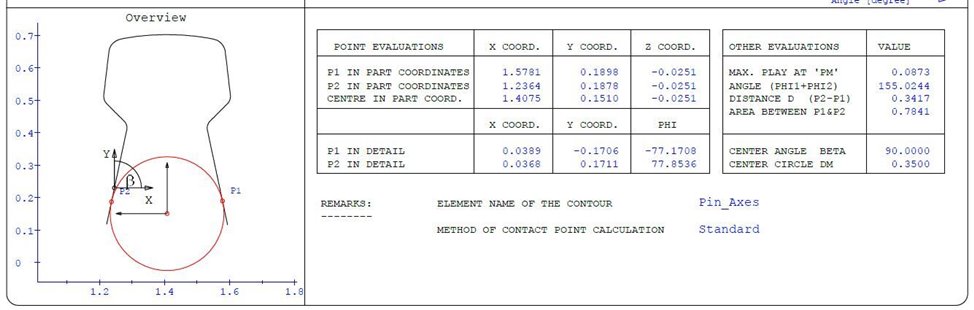
I did a test om a Cad model. You must insert your own values and element names.
CENTCIR (NAM=PROFIL, PLA=XY, DIA=100, ANG=180, SPT=4, PNT=P(1))
CENTCIR_P (NAM=PROFIL, RES=(CENT, PNT1, PNT2), PLA=XY, DIA=100)
!Calculate the distance to the centerpoint
DIPNTAXI (NAM=DIS(1), CSY=CSY(1), EL1=CENT, EL2=AXI(1), CPY=DEF$DIS1)
!Calculate the distance to the tangentpoint
DIPNTAXI (NAM=DIS(2), CSY=CSY(1), EL1=PNT2, EL2=AXI(2), CPY=DEF$DIS1)
!Add the radius off the centering ball
XY=DIS(1).$A+50
!Run DFNELE just one time
DFNELE (NAM=DIS(3), TYP=POI, CSY=CSY(1))
!Add the calculated realvalue to the element
PUTVAL (OBJ=DIS(3), DSC=X, TYP=ELE, VAL=XY)
!Evaluate
AddEva (NAM=DIS(3), EDT=N)
Hope this helps
Hello
I make another attempt to explain.
I explain in XY plane
First you calculate the distance to the center of the centering circle.
Make sure that the distance in Z betveen the axis and profil i close to zero, otherwise you have to prjekt the points (PRJPTS)
DIPNTAXI (NAM=DIS(1), CSY=CSY(1), EL1=CENT, EL2=AXI(1), CPY=DEF$DIS1)
DIPNTAXI (NAM=DIS(2), CSY=CSY(1), EL1=CENT, EL2=AXI(2), CPY=DEF$DIS1)
Get the result as an real value. It´s the A value från the element and it´s the same as DXYZ value from the evaluation above.
GETVAL (NAM=XY_DIS(1), OBJ=DIS(1), DSC=A, TYP=ELE)
GETVAL (NAM=XY_DIS(2), OBJ=DIS(2), DSC=A, TYP=ELE)
Take this values and add the radius for the centering cicle.
XY_DIS_RAD(1)=XY_DIS(1)+(0.35/2)
XY_DIS_RAD(2)=XY_DIS(2)+(0.35/2)
Define an element to put the calculated value in. That needs to be done only one time.
DFNELE (NAM=DIS(3), TYP=POI, CSY=CSY(1))
Put in the values that has been calculated as X and Y coordinates
PUTVAL (OBJ=DIS(3), DSC=X, TYP=ELE, VAL=XY_DIS_RAD(1))
PUTVAL (OBJ=DIS(3), DSC=Y, TYP=ELE, VAL=XY_DIS_RAD(2))
Evaluate the result in X and Y
AddEva (NAM=DIS(3), EDT=N)
| © 2025 Hexagon AB and/or its subsidiaries. | Privacy Policy | Cloud Services Agreement |